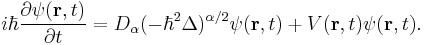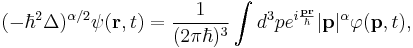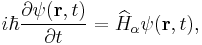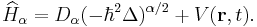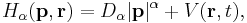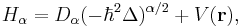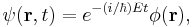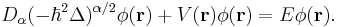Fractional Schrödinger equation
The fractional Schrödinger equation is a fundamental equation of fractional quantum mechanics. It was discovered by Nick Laskin (1999) as a result of extending the Feynman path integral, from the Brownian-like to Lévy-like quantum mechanical paths. The term fractional Schrödinger equation was coined by Nick Laskin[1]. The fractional Schrödinger equation has the following form [2]:
Here  is a 3-dimensional vector,
is a 3-dimensional vector, 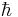 is the Planck constant,
is the Planck constant, 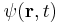 is the wavefunction, which is the quantum mechanical probability amplitude for the particle to have a given position
is the wavefunction, which is the quantum mechanical probability amplitude for the particle to have a given position  at any given time t,
at any given time t, 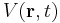 is a potential energy, and
is a potential energy, and  is the Laplace operator. Further,
is the Laplace operator. Further,  is a scale constant with physical dimension
is a scale constant with physical dimension ![[D_\alpha ]=\mathrm{erg}^{1-\alpha }\cdot \mathrm{cm}^\alpha \cdot \mathrm{sec}^{-\alpha }](/2012-wikipedia_en_all_nopic_01_2012/I/46788cfa534acdebc9a47a8b1ae9f73a.png) , (at α = 2, D2 =1/2m, where m is a particle mass), and the operator
, (at α = 2, D2 =1/2m, where m is a particle mass), and the operator 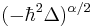 is the 3-dimensional fractional quantum Riesz derivative defined by
is the 3-dimensional fractional quantum Riesz derivative defined by
Here the wave functions in the space 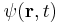 and momentum
and momentum 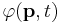 representations are related each other by the 3-dimensional Fourier transforms
representations are related each other by the 3-dimensional Fourier transforms
The index α in the fractional Schrödinger equation is the Lévy index, 1 < α ≤ 2. Thus, the fractional Schrödinger equation includes a space derivative of fractional order α instead of the second order (α = 2) space derivative in the standard Schrödinger equation. Thus, the fractional Schrödinger equation is a fractional differential equation in accordance with modern terminology[3]. This is the main point of the term fractional Schrödinger equation or a more general term fractional quantum mechanics[4]. At α = 2 fractional Schrödinger equation becomes the well-known Schrödinger equation.
The fractional Schrödinger equation has the following operator form
where the fractional Hamilton operator  is given by
is given by
The Hamilton operator,  corresponds to classical mechanics Hamiltonian function
corresponds to classical mechanics Hamiltonian function
where 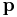 and
and  are the momentum and the coordinate respectively.
are the momentum and the coordinate respectively.
Time-independent fractional Schrödinger equation
The special case when the Hamiltonian 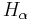 is independent of time
is independent of time
is of great importance for physical applications. It is easy to see that in this case there exist the special solution of the fractional Schrödinger equation
where 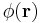 satisfies
satisfies
or
This is the time-independent fractional Schrödinger equation.
Thus, we see that the wave function 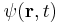 oscillates with a definite frequency. In classical physics the frequency corresponds to the energy. Therefore, the quantum mechanical state has a definite energy E. The probability to find a particle at
oscillates with a definite frequency. In classical physics the frequency corresponds to the energy. Therefore, the quantum mechanical state has a definite energy E. The probability to find a particle at  is the absolute square of the wave function
is the absolute square of the wave function 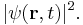 Because of time-independent fractional Schrödinger equation this is equal to
Because of time-independent fractional Schrödinger equation this is equal to  and does not depend upon the time. That is, the probability of finding the particle at
and does not depend upon the time. That is, the probability of finding the particle at  is independent of the time. One can say that the system is in a stationary state. In other words, there is no variation in the probabilities as a function of time.
is independent of the time. One can say that the system is in a stationary state. In other words, there is no variation in the probabilities as a function of time.
See also
- Fractional quantum mechanics
- Schrödinger equation
- Path integral formulation
- Relation between Schrödinger's equation and the path integral formulation of quantum mechanics
- Fractional calculus
References
- ^ N. Laskin, (2000), Fractional Quantum Mechanics and Lévy Path Integrals. Physics Letters 268A, 298-304.
- ^ N. Laskin, (2002), Fractional Schrödinger equation, Physical Review E66, 056108 [7 pages]. (also available online: http://arxiv.org/abs/quant-ph/0206098)
- ^ S. G. Samko, A. A. Kilbas, and O. I. Marichev, Fractional Integrals and Derivatives, Theory and Applications ~Gordon and Breach, Amsterdam, 1993
- ^ N. Laskin, (2000), Fractional Quantum Mechanics, Physical Review E62, 3135-3145. (also available online: http://arxiv.org/abs/0811.1769)
|
|||||